Sound slowness

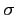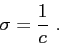The reciprocal of sound speed is present sistematically in the system given by Eq.(2.14). Such fact suggest that the system can be greatly simplified by defining the reciprocal of sound speed as a parameter of its own, called sound slowness $\sigma$:

$$\sigma = \frac{1}{c} .$$ (2.15)

With this new parameter the system Eq.(2.14) becomes

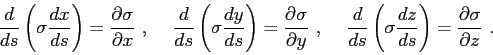$$\frac{d}{ds}\left( \sigma\frac{dx}{ds} \right) = \frac{\part... ...ac{dz}{ds} \right) = \frac{\partial{\sigma}}{\partial{z}} .$$ (2.16)

An additional simplification can be achieved by considering sound slowness as a vector parameter:

$$\mbox{\boldmath$\sigma$}= \left[ \sigma_x , \sigma_y , \sigma_z \right] .$$ (2.17)

Following this new definition each term inside parenthesis implies that

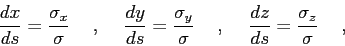$$\frac{dx}{ds} = \frac{\sigma_x}{\sigma} \hskip5mm , \hskip5m... ...hskip5mm \frac{dz}{ds} = \frac{\sigma_z}{\sigma} \hskip5mm ,$$ (2.18)

which transforms the system Eq.(2.16) into

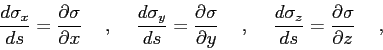$$\frac{d\sigma_x}{ds} = \frac{\partial{\sigma}}{\partial{x}} ... ...gma_z}{ds} = \frac{\partial{\sigma}}{\partial{z}} \hskip5mm ,$$ (2.19)

or, more compactly, in vector form:

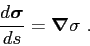$$\frac{d\mbox{\boldmath$\sigma$}}{ds} = \mbox{\boldmath$\nabla$}\sigma .$$ (2.20)